十項式排列 Decanomial Layout

Age: 4.75 & 7.75

Presentations:

• Tables Layout
• Decanomial Layout: Finding Squares
• Adjusted Decanomial: Commutative Law
• Adjusted Decanomial: Tower of Jewels
• Stacking the cubes

Even though I’ve shown Thumper presentations from squaring & cubing, and squares and cubes, we actually never finished some of the introductory exercises in squaring and cubing.  After reading a blog post about it from What Did We Do All Day, I finally found time to do it with the children on Sunday. What Did We Do All Day has a really great post about the exercises and also the variations within the different albums.  I don’t have the NAMC or Montessori R&D albums, though I do have another really detailed AMS album I used as reference when creating my math album this semester.  Ultimately, since my goal was just to show the children these exercises without accompanying written work, I went with  my album.  Partly because I feel I don’t have a strong grasp of how to implement followup work, but also partly because I just wanted Thumper to have the physical experience without all the written work, which often discourages her from working.

These presentations for me are just exercises that are fun and arouses the children’s interest in the squaring and cubing material, all the while giving them a sensorial experience of what it means to square and to cube.  I took all the related exercises together and we just flowed with it.  The whole thing took about 2-4 hours, with lots of breaks in between.  In the classroom, you would do this with at least two children.  I think 2 is a good number.  Anymore and the layouts get messed up way too easily.

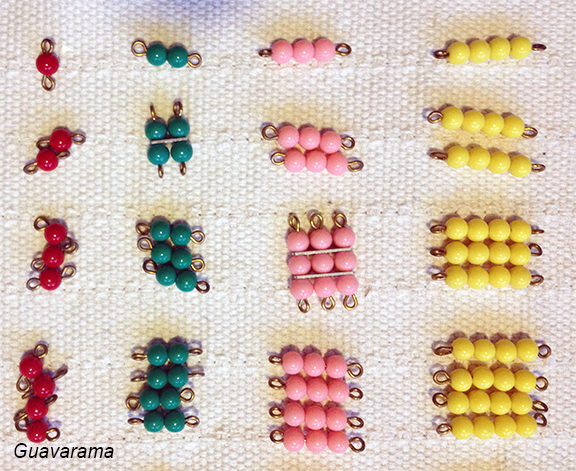The first presentation is the Tables Layout.  This is where you lay out the multiplication table with the color bead bars.  This took us the longest time and the kids got bored easily.  Here’s an instance where more than 2 kids would have been good.  I helped them line the bars up.  You really want them lined up well for the next exercises, so that they can see the patterns.  In my album, the tables are laid out from left to right, top to bottom. (1×1, 2×1, 3×1, 4×1….1×2, 2×2, 3×2).  My other album has a vertical layout and a horizontal layout, to emphasize the commutative property of the multiplication table.  At this point, Thumper already knows that and Astroboy is too young.  He just had fun counting and laying out the beads.  I had Thumper start on the top left end and Astroboy the bottom right end so they wouldn’t get in each other’s way.

After the tables are laid out, Thumper found the power scales  This is where you show them that 10=1²+9, 20=2²+16, etc.  If you find the difference between the second addend (9 & 16), and then find the difference between those, you get 2.  It’s just something interesting. Moving on to Second Decanomial layout: Finding Squares, you help them notice the squares in the layout and exchange them for the bead squares.  I told Astroboy that we were going to do some magic.  We circled our index finger over each potential-square, and said, “變變變!” (abracadabra).  Then we exchanged them for the bead square. At one point during all of our activities Astroboy said, “這個太好玩了！(This is so fun!)”  I was so happy that he super enjoyed the work for once.  I think it’s what propelled both of them to work on this for this many hours, till 9pm in fact. Once you find the squares, you now change the layout for Adjusted Decanomial: Commutative Law. I first pointed out to Astroboy that 1×2 = 2×1.  He did NOT get that.  Obviously not quite ready for multiplication.  But he could still help his sister exchange similar values.  For example, he would exchange 2 1-bars (1×2) for 1 2-bar (2×1) and 3 1-bar (1×3) for 1 3-bar (3×1).  You lay these bars vertically, as opposed to the original vertical layout of each bar.  When you’re done, you get this reflected L-shape for each number.  Basically by the end you have a row of golden beads on the bottom and a column of golden beads on the right as well.  Astroboy got very confused after we left the multiplication of 1.  So I had him exchange the 10s (1×10, 2×10, 3×10 for 10×1, 10×2, 10×3).  I was quite amazed I could point to a 7×10 and he knew, without counting, what the value was (70).  Since he knew, he could get 7 10-bars and exchange them. Oh, I forgot, even digging bead bars out of my Negative Snake Game set and my Colored Bead Stairs set, and my Intro to Golden Beads set, I still did not have enough bead bars for the adjusted decanomial layout.  You really need 2 sets of decanomial bead box, or the smaller checkerboard multiplication box in the classroom to do this. But we shouldered out, leaving some of the beads unexchanged.

Skipping over the Numerical Decanomial Layout presentation, we went right over to the culmination of these activities, the Tower of Jewels!   In this presentation, you exchange the bead bars for bead squares, and exchange those eventually for a bead cube.   I asked Astroboy (because I knew Thumper would get it too easily) by pointing out that the 3 square (三平方) is  3 taken 3 times (三，三次), does he see in the layout, others that are 3 taken 3 times?  He didn’t quite get it so I nudged in the the right direction.  Looking back, I think I needed to, as a teacher, to just sit back and let children think for awhile, not jump in after a few seconds of silence.  I read once that it takes children about 5 seconds after you ask them a question before they can process the question, think, and then reply.

You basically combine the loose bead bars of the same color to form squares.  Once I started with the “變變變!” on these bead bars, and showed them how stacking the bead squares form a bead cube that we can also exchange for, Thumper and Astroboy were so excited to do the work.   However, Thumper wanted to skip a step and just gather all the bead bars, and directly exchange them for a cube.  I had to stop her and ask her to exchange them for squares first.  I’m very glad I didn’t let her skip, because I realized one point of this lesson is to let them see that 9 9²  forms the 9-cube for example.  Sometimes their exchanges wouldn’t even out because they were fast and loose with their counting and exchange.  But it reminded them why we needed to carefully count.

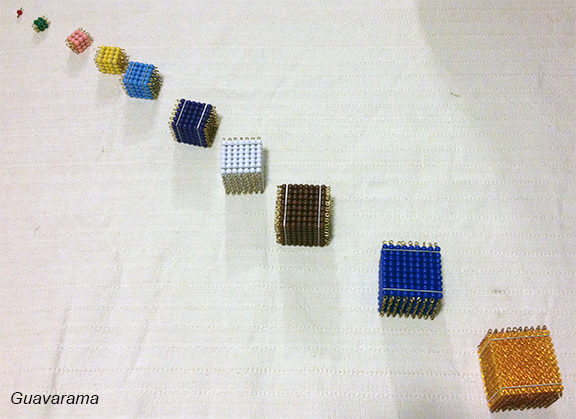
In the previous presentation, they were not able to exchange all the bead bars.  So, as we went along here, Thumper exchanged some bead bars for bead squares, and once those bead bars were available, we used them to finish the exchanges from the previous presentation. As the cubes started showing up, Astroboy noticed that they formed a stair case.  So after we were done, I let them play with this staircase for a bit, and then we stacked the cubes.  We’d stacked the cubes before to find their total.  In the album, finding the totals of cubes and squares is a game listed before the decanomial games.  But I don’t think there’s an actual specific order to how you need to present them.  So stacking the cubes is actually a really great and natural way to end this series of decanomial lessons.  We could have then founded the total of these cubes and see that the original tables layout gave you the same total value as a stacked cube layout.

So the Adjusted Decanomial: Commutative Law is actually called the Table of Pythagorus.  This is something the children also lay out in Primary.  I  need to find THAT work for Astroboy.  For Thumper, as followup, I’m going to see if she’ll be interested in laying this out on paper.  That’s the presentation we skipped.

Vocabulary

For both kids, especially Astroboy, we reviewed the vocabulary of squares and cubes.  He apparently did not really remember from his last lesson. I asked Thumper what their equivalent names are in English and found out that she’s thought squares were called square roots in English.  Also, in Chinese, you would add a 的 when you say 3 square.  So that it’s more like “3’s square”.

• square – 平方
• cube – 立方
• 3 square – 三的平方
• 3 cube – 三的立方
• decanomial –  十項式
• Table of Pythagorus – 十项式平方