When you homeschool, sometimes Saturdays and Sundays look just like a regular day, especially when Baba is sleeping in and no-one’s there to play with the children.
This morning, the kids quietly “played” in the classroom while I worked on my album. Astroboy saw the small bead frame laying around, placed it on his lap, and started counting the beads to himself. He worked on the frame 1-2 months ago for about 10 minutes. When I heard him still counting away after 5 minutes, I walked over to see what was up. He was excited when I pointed out that he had a seven-zero-zero on the frame. Seeing an opportunity, I told him that he needs to work on a mat and I’ll show him other numbers.
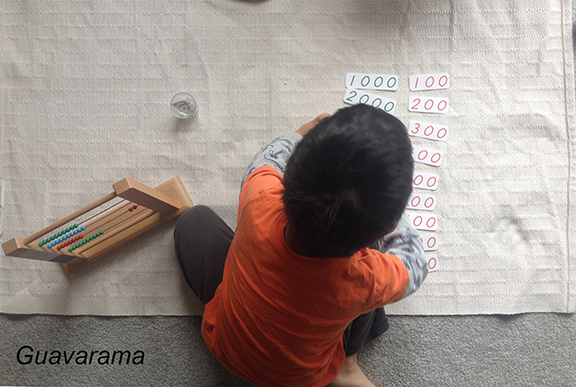
After pulling out a rug and small numeral cards, we laid them out together. I then pulled out 700, put it beneath the frame, read 700 on the card and pointed out 7-0-0 on the frame. “Oooo!” He said. He saw a connection and was excited.
What’s the Small Bead Frame?
The small bead frame is one of the last steps in moving to abstraction in Primary class for math. Here, the value of 1 bead can be different depending on its color and placement in the frame. In the material before this, the Stamp Game, the values of the stamps are printed on the stamp (1 vs 10 vs 100 vs 1000), they’re not all printed 1.
The frame is almost like an abacus. The four rows are, from top to bottom, green (1-10), blue (10-90), red (100-900), and green (1000-9000). The colors repeat because of how we name numbers; simple family, thousands, millions, etc. It’s funky cuz in Chinese the counter goes up to 1000 before it starts repeating. So in Chinese, Astroboy actually likes to count up to 10,000.
You use the bead frame to add and subtract numbers up to 4 digit numbers. I think you can multiply too but we typically use the Large Bead Frame to do that, as that goes up to 1 million. Montessori math doesn’t teach operations with 1 digit, then 2 digit, then 3 digit. You do have other materials that will work with 1 digit, but it’s in the memorization thread of the curriculum. Whereas this material is part of learning the process of operations.
As for the presentation itself, you start with introduction to how to read numbers on the frame. You can slowly count from 1 to 9999 to get a sense of how you exchange 10 green beads, then you can practice reading and composing numbers, and then lastly do the addition/subtraction.
What We Did Today
Though Astroboy is technically not ready to use this material, he really likes it because he really likes to count. That’s what we used it for the last time we used the material. He wanted to move the beads and count them one by one; whereas Thumper did not want to do that. I always like to see how different age children will respond to the same material. Since the material can be used for many years, all you do is adjust the presentation difficulty.

In any case, I knew that Atroboy needed more practice composing the numbers. So I told him that I wanted to show him something interesting. Closing my eyes, I circled over the hards with my index finger and picked out 4 numbers, one from each category. We slid the cards together (children love this for some reason) and composed the number 5946. I then showed him how to count out the beads, starting from the units place. 1,2,3,4,5,6; 10, 20, 30, 40, etc.
It’s important to note that I’m counting their actual values. I’m not counting 1, 2, 3 when I’m counting the 100’s beads. I’ve noticed how my write-ups tends to have the teacher say the actual value rather than abstract counting. The presentations emphasize the actual value of a number. I feel like I skipped this step pretty quick when I learned how my operations in elementary school. For example, when we add 15+35 vertically, we are taught to add 1 and 3. We don’t learn we’re adding 10 and 30. And then you’ll see worksheets that tries to make sure children have this number sense, that a 10 is actually 10 1’s instead of a 1 followed by a 0.
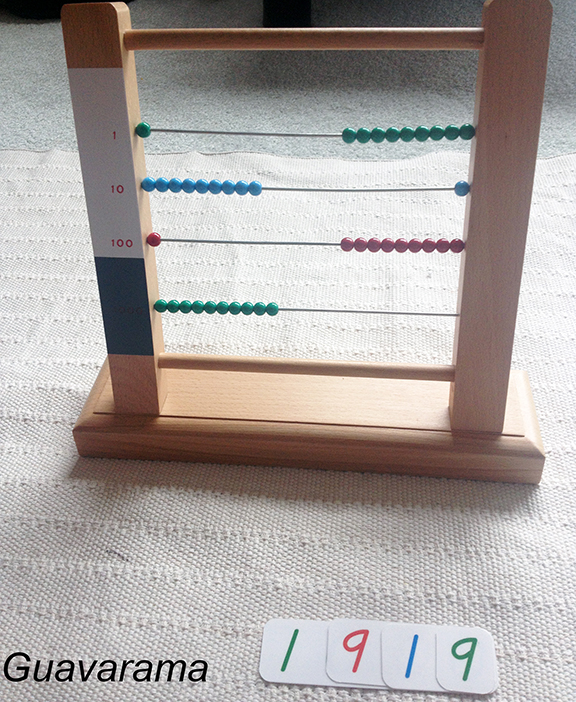
After 5946, I told him I wanted to compose a funny number and picked 1919. Thumper, who was watching us, could see the pattern; that the beads form 1919 on one side and 9191 on the other. Astroboy didn’t catch on. It was VERY HARD to hold my tongue, but I did.

However, Astroboy was still very excited by these funny numbers and wanted to do it himself. So he pulled out 1111 and 9999 and giggled and giggled how he could compose two numbers at the same time. This then lead to him stacking all the cards in similar order, 1111, 2222, 3333,….9999.
He went through and counted 9999, put the number on his “train”, then 8888, put that on his train, etc till 1111. Then he took his train for a ride. This took probably him 15-30 minutes. I have a picture of him super intensely concentrating on composing one number, before wiggling around and futzing with his train again, and the back to concentrating. The room would get really quiet while he was doing that. It was a real joy to watch.
Normalization
I recorded him a bit. I felt like I was observing a child in one of my 2 hour observations in a Montessori classroom. The kids wiggle and can’t stop moving often, but there is quietness and intentional movement when they’re concentrating and really working on something. Often they also obsess over details like lining things up just right.
If I saw a child like this in a 3-6 classroom, I would say he’s not quite normalized yet but he’s getting there. In our philosophy class, we learn that normalization is basically when they’re doing concentrated work. It’s just the degree of concentration. When they are very normalized, they have long periods of purposeful movement in the classroom.
He was constantly stopping when he got distracted by what his sister was doing. BUT, he did go back and finished everything. There are children at the beginning of the normalization process, who will get distracted by the other thing, and move on to another work, or just stand at another child’s desk for 10 minutes, talking. In a classroom of 40 children, it’s easier for the teacher not to get involved and tell the student to focus and go back to work. I had to really work on that as a teacher today, to not disrupt him while he’s working and let him be; to not remind him to go back to work. I think I knew to do this partly because there would be a period of super quietness when he was concentrating on counting out his numbers.
All in all really good work today. I’ve been much better about how I work in the classroom after my observation at a local Motnessori elementary school. More about that in another post.
